Gerrit Großmann

---

Research

Update: I am currently working on the NextAID research program.

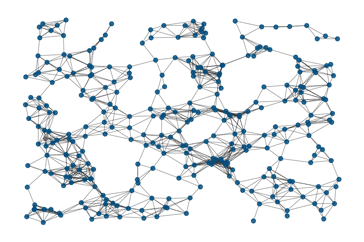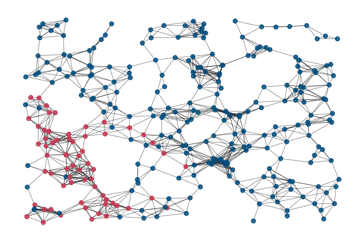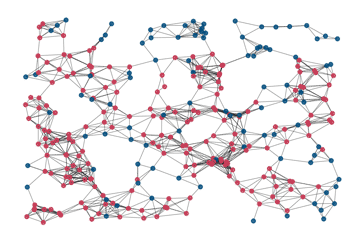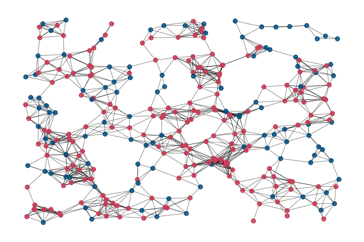
I am working on numerical methods (like fast simulations or model reduction techniques) for the analysis of stochastic dynamical processes unfolding on complex networks. My research aims at understanding how stochastic interactions, that are constrained by a network topology, shape collective emerging phenomena such as epidemics, rumors, blackouts in power grids, or burst in biological neural networks. The image shows a continuous-time SIS contagion process (infected nodes in red, healthy nodes in blue) on a geometric network.

Tutorials

• Spreading Dynamics in Complex Networks at the 20th Conference on Measurement, Modelling and Evaluation of Computing Systems (MMB), 2020 - Cancelled due to COVID-19 :(.

Tools

• LumPy
• NetworkEpidemicPlayground (browser-based)
• More tools on Github.

Teaching

• Deep Generative Diffusion Models (WS 22/23)
• Deep Learning for Drug Discovery (SoSe 22)
• Concurrent Programming (SoSe 21)
• Exploring Graph Neural Networks (SoSe 21)
• Statistics Lab (SoSe 20)
• Exploring Complex Network Dynamics (WS 19/20)
• Probabilistic Models and Data Analysis (SoSe 19)
• Ringvorlesung: Perspektiven der Informatik (WS 18/19)
• Probabilistic Models of Concurrency (WS 18/19)
• Pattern and Speech Recognition

Opinion Pieces

• 7 Reasons Not to Use ODEs for Epidemic Modeling
• Der Nutzen von Corona-Modellen bleibt ungeklärt (The usefulness of corona models remains uncertain), Saarbrücker Zeitung; Feb., 4th, 2022

Co-supervised Students

• Jonas Klesen, Research Immersion Lab, 2020.
  Learning vaccine allocation strategies to control epidemic outbreaks on networks
• Lisa Heidmann, Bachelor thesis, 2020.
  Effects of Interventions on the COVID-19 Outbreak: A Network-based Approach
• Julian Zimmerlin, Bachelor thesis, 2021.
  Learning Dynamical Processes to Infer the Underlying Network Structure
• Yan Yan Lau, Bachelor thesis, 2022.
  Adapting the Approximate Master Equations for Realistic Epidemic and Network Dynamics

Publications

• G. Großmann, J. Zimmerlin, M. Backenköhler, V. Wolf: GINA: Neural Relational Inference From Independent Snapshots, Preprint, 2021.
• M. Backenköhler, L. Bortolussi, G. Großmann, V. Wolf: Abstraction-Guided Truncations for Stationary Distributions of Markov Population Models
• G. Großmann, M. Backenköhler, V. Wolf: Heterogeneity matters: Contact structure and individual variation shape epidemic dynamics, PLOS ONE, 2021, Preprint PDF.
• G. Großmann, M. Backenköhler, V. Wolf: Epidemic Overdispersion Strengthens the Effectiveness of Mobility Restrictions, MedRxiv, Poster Abstract, 2021.
• G. Großmann, L. Bortolussi, V. Wolf: Efficient Simulation of non-Markovian Dynamics on Complex Networks, PLOS one, PDF
• M. Backenköhler, L. Bortolussi, G. Großmann, V. Wolf: (TACAS 2021) Analysis of Markov Jump Processes under Terminal Constraints
• G. Großmann, M. Backenköhler, J. Klesen, V. Wolf: Learning Vaccine Allocation from Simulations, The 9th International Conference on Complex Networks and their Applications, 2020. Preprint PDF
• G. Großmann, M. Backenköhler, V. Wolf: Importance of Interaction Structure and Stochasticity for Epidemic Spreading: A COVID-19 Case Study, QEST 2020.
• G. Großmann, M. Backenköhler: (Poster Abstract) Birth-Death Processes Reproduce the Infection Footprint of Complex Networks, HSB 2020.
• G. Großmann, L. Bortolussi, V. Wolf: Rejection-Based Simulation of Non-Markovian Agents on Complex Networks, The 8th International Conference on Complex Networks and their Applications, 2019.
• G. Großmann, L. Bortolussi: Reducing Spreading Processes on Networks to Markov Population Models, QEST 2019, PDF.
• G. Großmann, V. Wolf: Rejection-Based Simulation of Stochastic Spreading Processes on Complex Networks HSB 2019, PDF.
• C. Kyriakopoulos, G. Großmann, V. Wolf, L. Bortolussi: Lumping of Degree-Based Mean Field and Pair Approximation Equations for Multi-State Contact Processes, Physical Review E, 2018.
• G. Großmann, C Kyriakopoulos, L Bortolussi, V Wolf: Lumping the Approximate Master Equation for Multistate Processes on Complex Networks, QEST 2018, PDF.

Theses by G. Großmann:

• PhD Dissertation Thesis: Stochastic Spreading on Complex Networks (Review version avaliable on Github)
• Master’s Thesis: Lumping the Approximate Master Equation for Stochastic Processes on Complex Networks (avaliable upon request or at Campus-Bibliothek).
• Bachelor’s Thesis: Efficient Computation of Likelihoods in Large Markov Models (avaliable upon request or at Campus-Bibliothek).